Enter 1 out of 3 to solve for the other 2 missing sides Special right triangle 30 60 90 is one of the most popular right triangles The 30 60 90 right triangle is a special case triangle with angles measuring 30 60 and 90 degrees If you want to read more about that special shape check our calculator dedicated to the 30 60 90 triangleThe main rule of triangles is that it has one right angle and while the other two angles each measure 45° 45 ° The lengths of the sides adjacent to the right triangle, the shorter sides have an equal length Another rule is that the two sides of the triangle or legs of the triangle that form the right angle are congruent in length Special Right Triangles Formula Triangle Let's repeat the same procedure for the special right triangle {eq}30^{\circ} 60^{\circ} 90^{\circ} {/eq} Consider the following image of
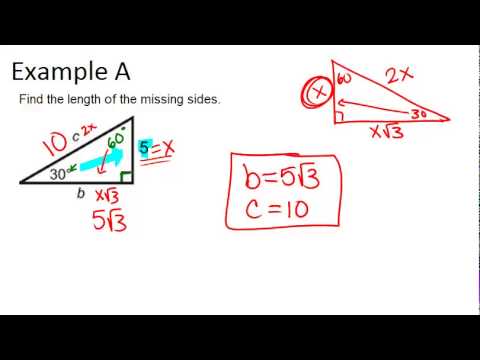
30 60 90 Right Triangles Examples Geometry Concepts Youtube
Formula for sides of a 30 60 90 triangle
Formula for sides of a 30 60 90 triangle-Before understanding formulas, let us first recall what is a triangle is one of the special right triangles where the three interior angles measure 30°, 60°, and 90° Right triangles with interior angles are known as special right triangles ie, their sides and angles are predictable and consistent The legs of such a triangle are equal, the hypotenuse is calculated immediately from the equation c = a√2 If the hypotenuse value is given, the side length will be equal to a = c√2/2 Triangles (set squares) The red one is the 45 45 90 degree angle triangle



A 30 60 90 Triangle
Correct answer Explanation We know that in a 3060=90 triangle, the smallest side corresponds to the side opposite the 30 degree angle Additionally, we know that the hypotenuse is 2 times the value of the smallest side, so in this case, that is 10 The formula forWhat I want to do in this video is discuss a special class of triangles called triangles and I think you know why they're called this the measures of its angles are 30 degrees 60 degrees and 90 degrees and what we're going to prove in this video this tends to be a very useful result at least for a lot of what you see in a geometry class and then later on in trigonometry class is theThis is a triangle whose three angles are in the ratio 1 2 3 and respectively measure 30° (π / 6), 60° (π / 3), and 90° (π / 2)The sides are in the ratio 1 √ 3 2 The proof of this fact is clear using trigonometryThe geometric proof is Draw an equilateral triangle ABC with side length 2 and with point D as the midpoint of segment BC
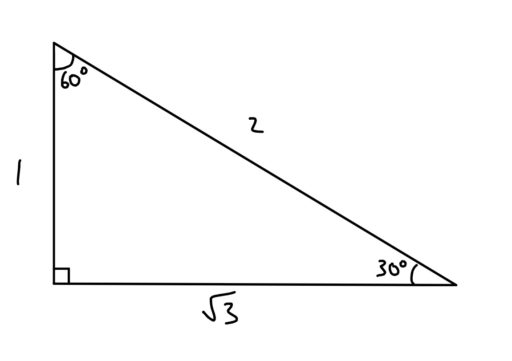
A 30 60 90 triangle completes an arithmetic progression 3030=6030 =90 An arithmetic progression is a sequence of numbers in which the difference of any two successive numbers is a constant For instance, 2,4,6,8 is an arithmetic progression with a constant of 2Special Triangle Relationships Triangles A triangle is a right triangle whose internal angles are 30, 60 and 90 degrees The three sides of a triangle have the following characteristics All three sides have different lengths The shorter leg, b, is half the length of the hypotenuse, c That is, b=c/2See also Side /angle relationships of a triangle In the figure above, as you drag the vertices of the triangle to resize it, the angles remain fixed and the sides remain in this ratio Corollary If any triangle has its sides in the ratio 1 2 √3, then it is a triangle
A triangle is a special right triangle whose angles are 30º, 60º, and 90º The triangle is special because its side lengths are always in the ratio of 1 √32 What is the formula for a 45 45 90 Triangle?A right triangle (literally pronounced "thirty sixty ninety") is a special type of right triangle where the three angles measure 30 degrees, 60 degrees, and 90 degrees The triangle is significant because the sides exist in an easytoremember ratio 1 √3 3 2 That is to say, the hypotenuse is twice as long as the shorter leg, and The sides of a right triangle lie in the ratio 1√32 The side lengths and angle measurements of a right triangle Credit Public Domain We can see why these relations should hold by plugging in the above values into the Pythagorean theorem a2 b2 = c2 a2 ( a √3) 2 = (2 a) 2 a2 3 a2 = 4 a2




30 60 90 Triangle Theorem Ratio Formula Video
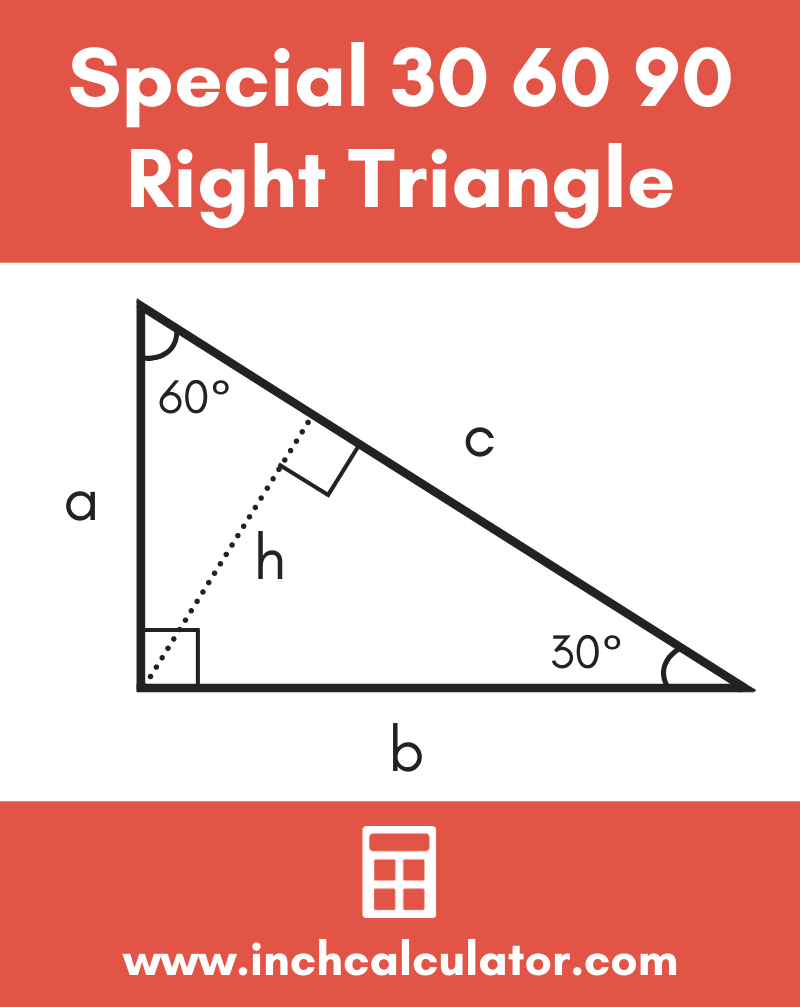



30 60 90 Special Right Triangle Calculator Inch Calculator
Answer (1 of 3) If we take a triangle ABC, right angled at A And assume < B = 60°, < C = 30° Then, sin 60° = opposite side / hypotenuse => √3/2 = AC/BC = √3x / 2x & By pythagoras law, BC² = AB² AC² => BC² = x² 3x² = 4x² => BC = 2x Hence, ratio of the lengths of ABACBC = 1 √3 2 Since the right angle is always the largest angle, the hypotenuse is always the longest side using property 2 We can use the Pythagorean theorem to show that the ratio of sides work with the basic triangle above a2b2=c2 12(3–√)2=13=4=c2 4–√=2=cThe triangle is one example of a special right triangle It is right triangle whose angles are 30°, 60° and 90° The lengths of the sides of a triangle are in the ratio of 1√32 The following diagram shows a triangle and the ratio of the sides Scroll down the page for more examples and solutions on how to use




Learn About The 30 60 90 Triangle Caddell Prep Online



Appendix All The Formulas
If the shortest side of a triangle is 5sqrt(3) units long, find the length of the side that is opposite the 60 degree angle A symmetrical canyon isSolution This is a 30°60°90° triangle and we know that these triangles have the proportions 12√3 The side that measures 5 units corresponds to the 30° angle and the side we are looking for corresponds to the 90° angle Therefore, its proportion is 2 and we haveArea of a Triangle The formula to calculate the area of a triangle is = (1/2) × base × height In a rightangled triangle, the height is the perpendicular of the triangle Thus, the formula to calculate the area of a rightangle triangle is = (1/2) × base × perpendicular



1



5 5 Special Triangles
What are the sides of a Triangle? A $$ is one of the must basic triangles known in geometry and you are expected to understand and grasp it very easily In an equilateral triangle, angles are equal As they add to $180$ then angles are are all $\frac {180}{3} = 60$ And as the sides are equal all sides are equal (see image) So that is a $$ triangle30 ° 60 ° 90 ° Triangles A 30 °− 60 °− 90 ° triangle is commonly encountered right triangle whose sides are in the proportion 1√32 The measures of the sides are x, x√3, and 2x




30 60 90 Triangle Definition Theorem Formula Examples




30 60 90 Right Triangles Examples Geometry Concepts Youtube
In 30 60 90 triangle the ratios are 1 2 3 for angles (30° 60° 90°) 1 √3 2 for sides (a a√3 2a)Of all these special right triangles, the two encountered most often are the 30 60 90 and the 45 45 90 triangles For example, a speed square used by carpenters is a 45 45 90 triangle In the day before computers when people actually had to draw angles, special tools called drawing triangles were used and the two most popular were the 30 60 90 A triangle is a right triangle with angle measures of 30º, 60º, and 90º (the right angle) Because the angles are always in that ratio, the sides are also always in the same ratio to each other The side opposite the 30º angle is the shortest and the length of it is usually labeled as x The side opposite the 60º angle has a



A 30 60 90 Triangle



1
A triangle is a special right triangle (a right triangle being any triangle that contains a 90 degree angle) that always has degree angles of 30 degrees, 60 degrees, and 90 degrees Because it is a special triangle, it also has side length values which are always in a consistent relationship with one anotherA triangle is a right triangle with angle measures of 30º, 60º, and 90º (the right angle) Because the angles are always in that ratio, the sides areHere is the proof that in a 30°60°90° triangle the sides are in the ratio 1 2 It is based on the fact that a 30°60°90° triangle is half of an equilateral triangle Draw the equilateral triangle ABC Then each of its equal angles is 60° (Theorems 3 and 9) Draw the straight line AD bisecting the angle at A into two 30° angles




30 60 90 Triangle Rules Sides Ratio Of A 30 60 90 Triangle Video Lesson Transcript Study Com




30 60 90 Triangle Theorem Ratio Formula Video
A triangle where the angles are 30°, 60°, and 90° If you draw an altitude in an equilateral triangle, you will form two congruent 30º 60º 90º triangles Starting with the sides of the equilateral triangle to be 2, the Pythagorean Theorem will allow us to establish pattern relationships between the sides of a 30º 60º 90º triangleIn any triangle, you see the following The shortest leg is across from the 30degree angle, the length of the hypotenuse is always double the length of the shortest leg, and you can find the length of the long leg by multiplying the short leg by the square root of 3Special Triangles Isosceles and Calculator This calculator performs either of 2 items 1) If you are given a right triangle, the calculator will determine the missing 2 sides Enter the side that is known After this, press Solve Triangle 2) In addition, the calculator will allow you to same as Step 1 with a right triangle



How To Work With 30 60 90 Degree Triangles Education Is Around



30 60 90 Triangle Formulas Rules And Sides Science Trends
Special right triangles hold many applications in both geometry and trigonometry In this lesson you will learn the general formula for the ratios, and how to find missing sides of any 30 60 90 right triangleRight triangle calculator, 30 60 90 formula, 45 triangle, special area, unit circle calculatorWatch more videos on http//wwwbrightstormcom/math/geometrySUBSCRIBE FOR All OUR VIDEOS!https//wwwyoutubecom/subscription_center?add_user=brightstorm2VI




Special Right Triangles Formulas 30 60 90 And 45 45 90 Special Right Triangles Examples Pictures And Practice Problems




30 60 90 Triangle Theorem Ratio Formula Video
Proving the ratios between the sides of a triangleWatch the next lesson https//wwwkhanacademyorg/math/geometry/right_triangles_topic/special_rigA triangle having angles of 30° , 60° , 90° is a right angled triangle Consider a triangle ABC, with angle A =90° , angle B= 30° and angle C =90° Let a, b and c be the sides opposite A, B and C respectively Then, a²=b²c² and b=aCos30°=a√2 andA triangle is a special right triangle that contains internal angles of 30, 60, and 90 degrees Once we identify a triangle to be a 30 60 90 triangle, the values of all angles and sides can be quickly identified Imagine cutting an equilateral triangle vertically, right down the middle Each half has now become a 30 60 90 triangle




A Full Guide To The 30 60 90 Triangle With Formulas And Examples Owlcation




30 60 90 Triangle Theorem Ratio Formula Video
30 60 90 triangle sides calculator If you just know the length of one "leg" of a 45 45 90 triangle (figure 8), multiply it by the square root of 2 (about 1414) to obtain the hypotenuse length Clicking "RESET" clears all of the boxes Triangles are classified as "special right triangles" Calculate the cyclist's average speed in mph A triangle is a right triangle with angle measures of 30º, 60º, and 90º (the right angle) Because the angles are always in that ratio, the sides are also always in the same ratio to each other The side opposite the 30º angle is the shortest and the length of itThe reason these triangles are considered special is because of the ratios of their sides they are always the same!




30 60 90 Triangle Calculator Formula Rules




30 60 90 Triangle Theorem Ratio Formula Video
Right Triangle Calculator Although all right triangles have special features – trigonometric functions and the Pythagorean theorem The most frequently studied right triangles , the special right triangles, are the 30, 60, 90 Triangles followed by the 45, 45, 90 triangles




How To Use The Special Right Triangle 30 60 90 Studypug
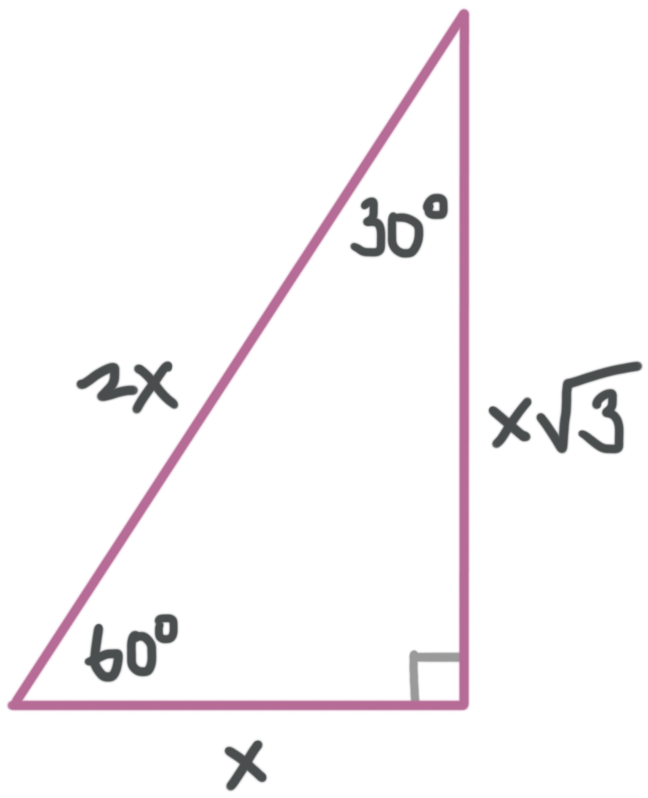



How To Solve 30 60 90 Triangles Krista King Math Online Math Tutor




A Full Guide To The 30 60 90 Triangle With Formulas And Examples Owlcation




30 60 90 Triangle Theorem Ratio Formula Video




30 60 90 Triangle Theorem Ratio Formula Video




Math Theorem Geometry Flashcards Quizlet




30 60 90 Formula Learn Formula For Calculating The 30 60 90 Measures




30 60 90 Triangle Theorem Properties Formula Video Lesson Transcript Study Com




Relationships Of Sides In 30 60 90 Right Triangles Ck 12 Foundation




What Is A 30 60 90 Degree Triangle Virtual Nerd Can Help




Relationships Of Sides In 30 60 90 Right Triangles Ck 12 Foundation




A Quick Guide To The 30 60 90 Degree Triangle Dummies




How To Find The Sides Of A 30 60 90 Right Triangle Math Wonderhowto
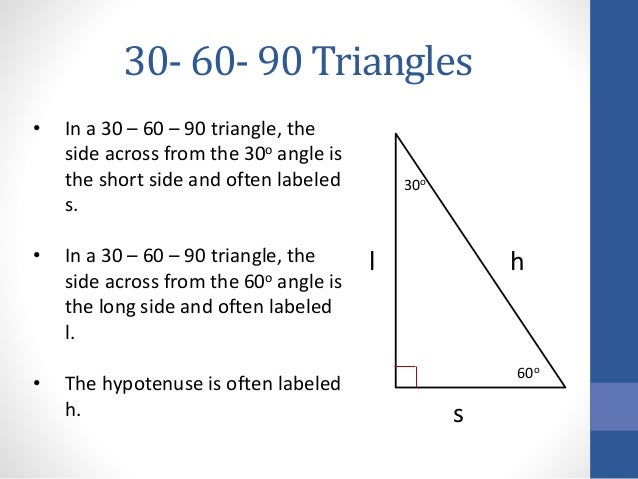



30 60 90 Triangles
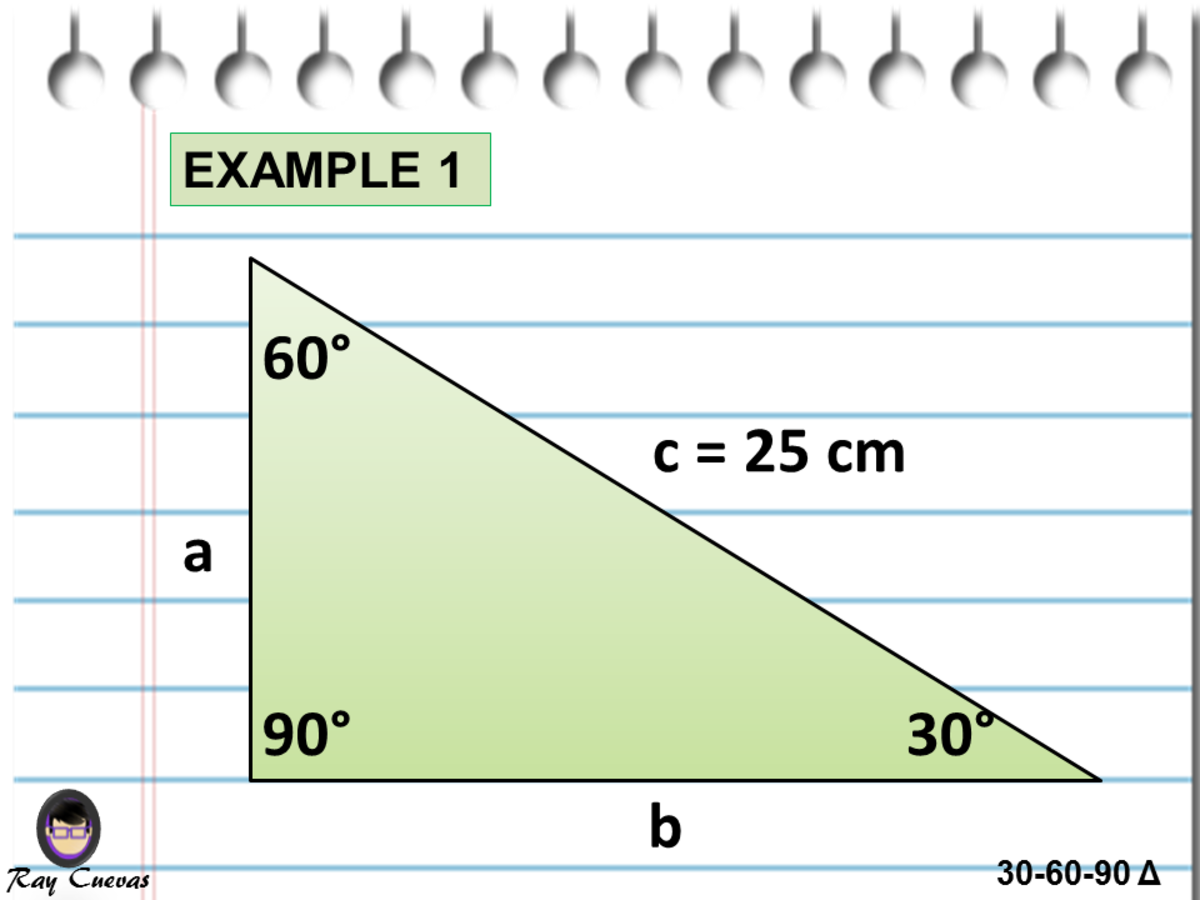



A Full Guide To The 30 60 90 Triangle With Formulas And Examples Owlcation




30 60 90 Triangle Theorem Properties Formula Video Lesson Transcript Study Com




30 60 90 Triangle Theorem Ratio Formula Video
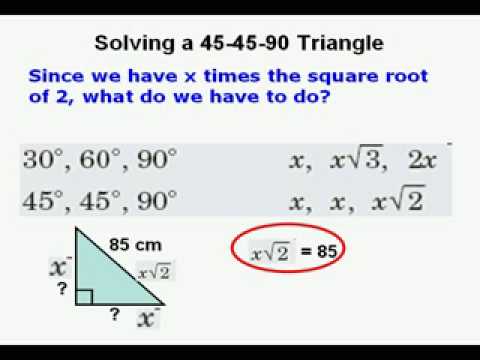



Find Sides Of 45 45 90 30 60 90 Right Triangles Youtube




30 60 90 Triangle Explanation Examples



45 45 90 And 30 60 90 Triangles Zona Land Education



1




Special Right Triangles Chapter 7 4 Special Right Triangles Triangles Triangles Ppt Download
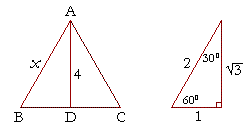



The 30 60 90 Triangle Topics In Trigonometry




30 60 90 Triangle Formulas Rules And Sides Science Trends



The Easy Guide To The 30 60 90 Triangle




A Quick Guide To The 30 60 90 Degree Triangle Dummies
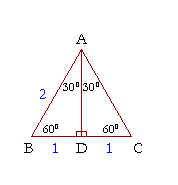



30 60 90 Right Triangle Side Ratios Expii




30 60 90 Triangle Definition Theorem Formula Examples



1
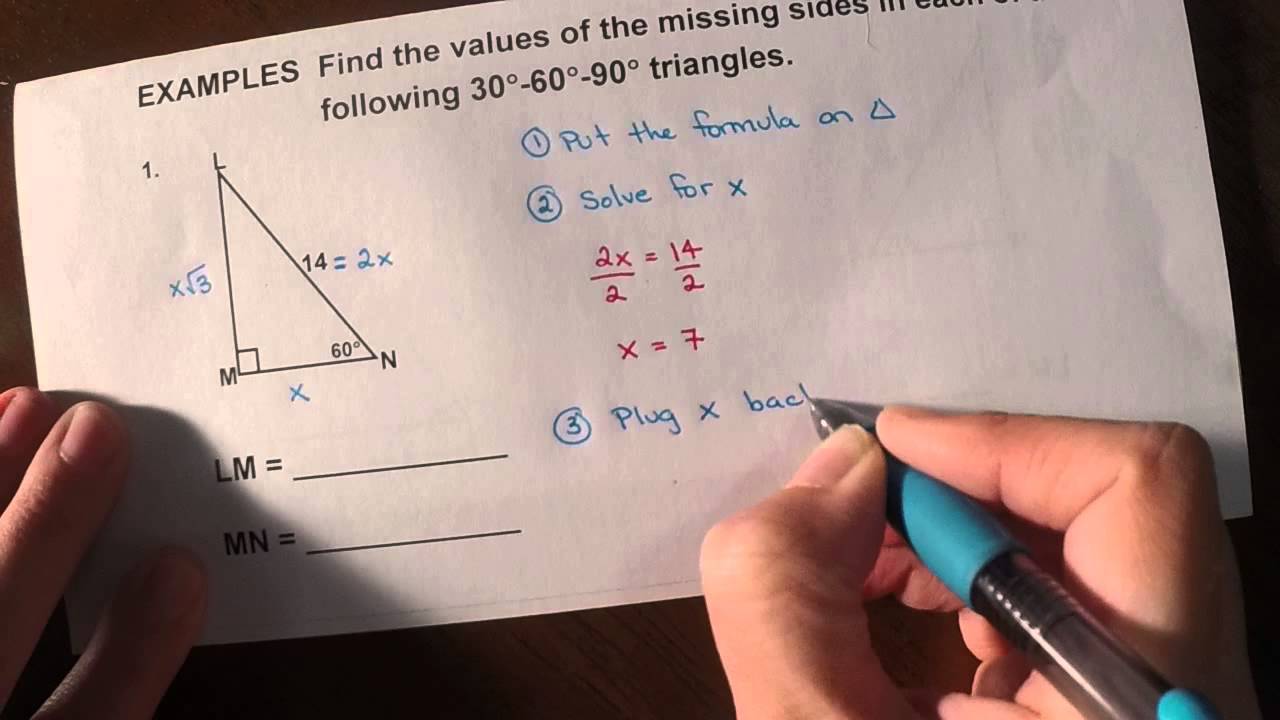



30 60 90 Triangles Youtube




Special Right Triangles 30 60 90 And 45 45 90 Triangles Youtube




How To Work With 30 60 90 And 45 45 90 Degree Triangles Dummies



30 60 90 Triangle Rules




30 60 90 Right Triangle Side Ratios Expii



The Easy Guide To The 30 60 90 Triangle




The Complete Guide To The 30 60 90 Triangle




30 60 90 Triangle Rules Sides Ratio Of A 30 60 90 Triangle Video Lesson Transcript Study Com




Relationships Of Sides In 30 60 90 Right Triangles Ck 12 Foundation




Instructions Use The Ratio Of A 30 60 90 Triangle To Solve For The Variables Make Sure To Simplify Brainly Com




30 60 90 Special Right Triangles Youtube
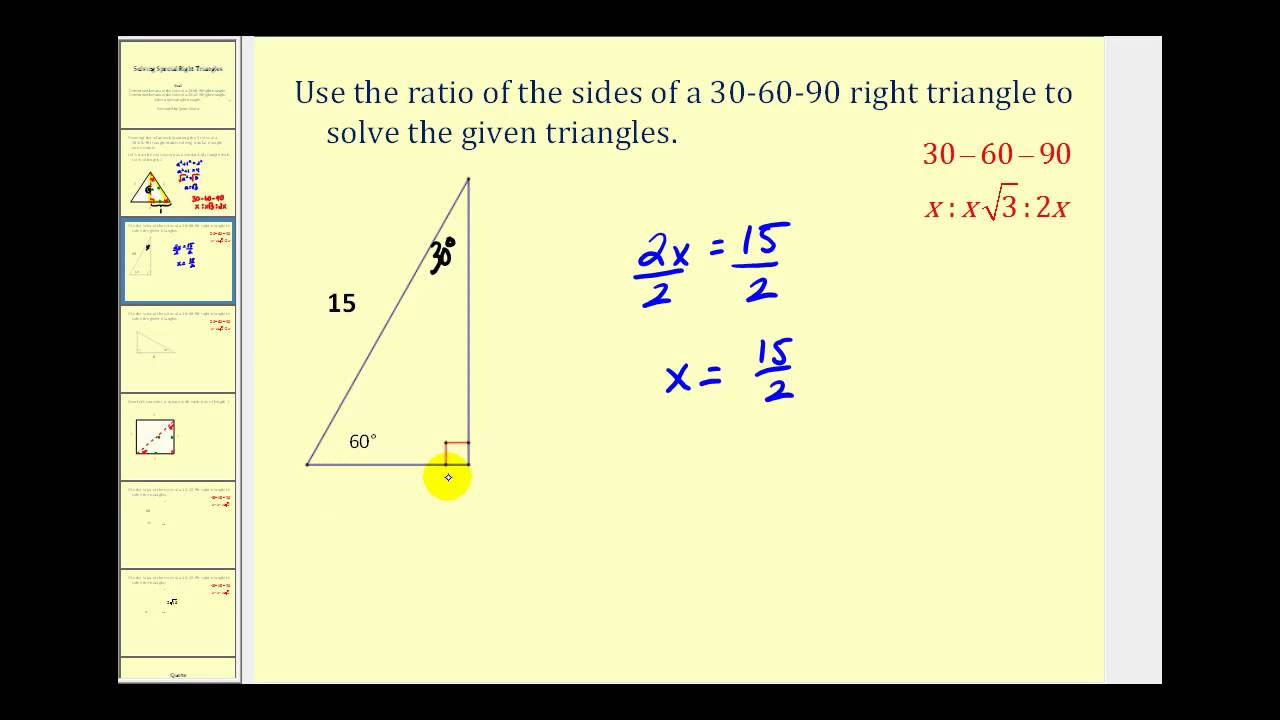



Solving Special Right Triangles Youtube




Special Right Triangles Fully Explained W 19 Examples




How To Find The Sides Of A 30 60 90 Right Triangle Math Wonderhowto
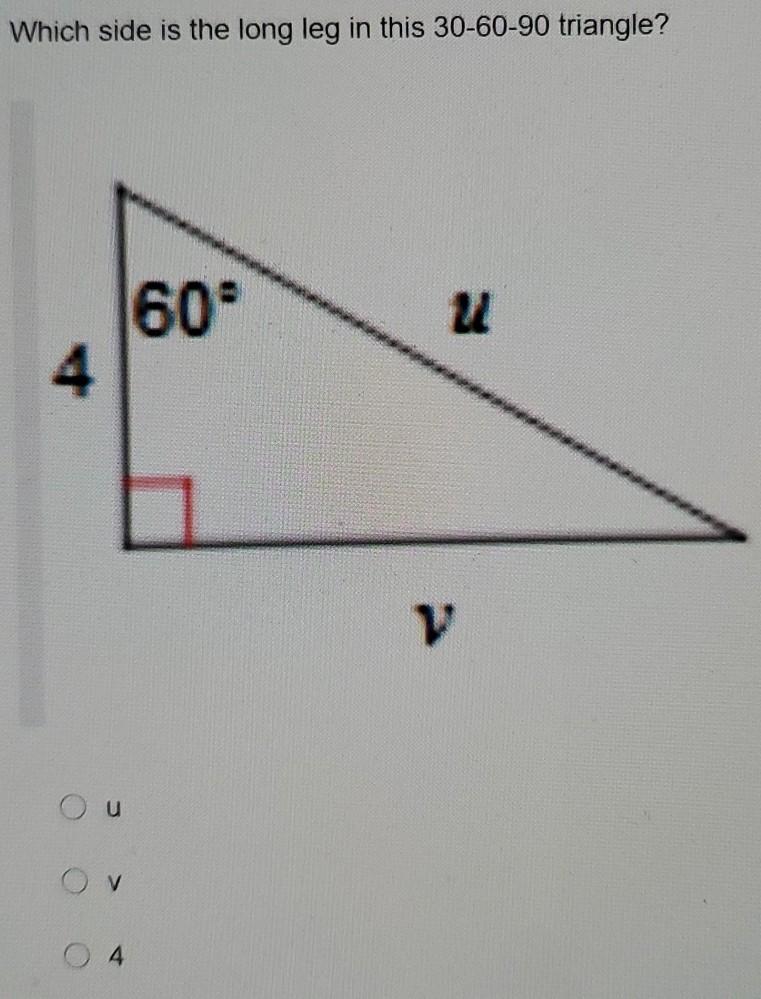



Solved Which Side Is The Long Leg In This 30 60 90 Triangle Chegg Com



Solve A 30 60 90 Triangle With Gradea
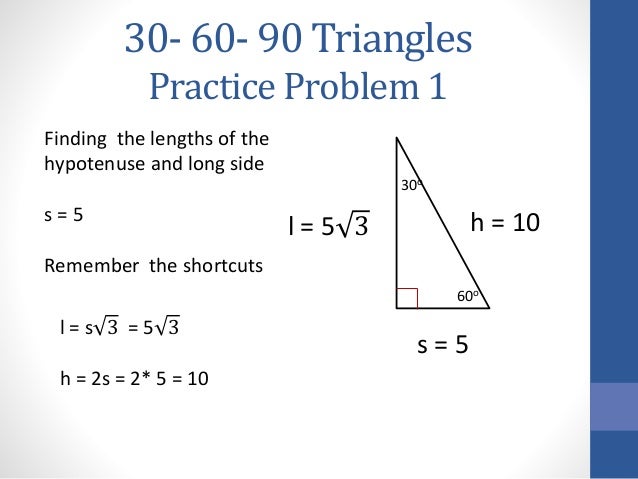



30 60 90 Triangles




Solved Deriving The Ratio Of The Sides Of A 30 60 90 Chegg Com




30 60 90 Right Triangles Free Math Help



The Easy Guide To The 30 60 90 Triangle




To Remember 30 60 90 Triangles One Boy Has An X S One Boy Has 2 X S And The Middle Boy Had 3 X S That He S Still Hung Teaching Materials Teaching Remember
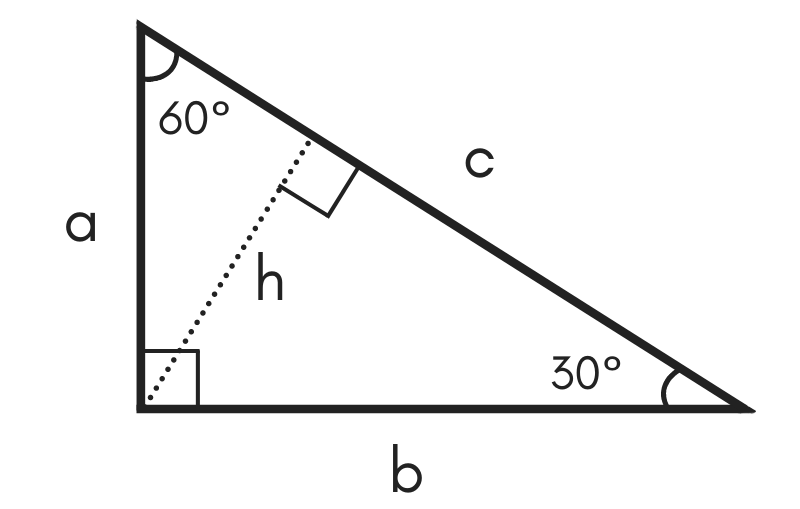



30 60 90 Special Right Triangle Calculator Inch Calculator



The Easy Guide To The 30 60 90 Triangle
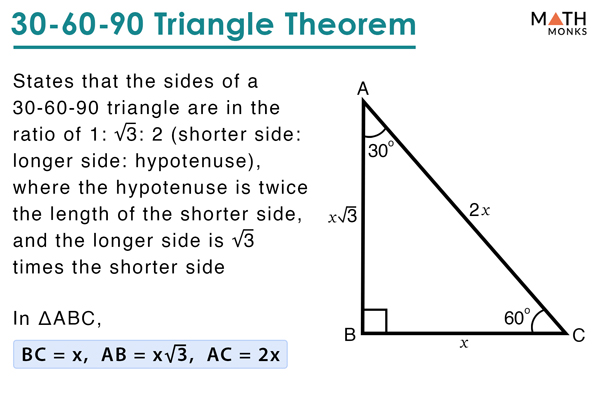



30 60 90 Triangle Definition Formulas Examples




30 60 90 Right Triangles Solutions Examples Videos




30 60 90 Triangle Calculator Formula Rules




Finding The Missing Side Of 30 60 90 Triangle Youtube




30 60 90 Triangle Calculator Formula Rules
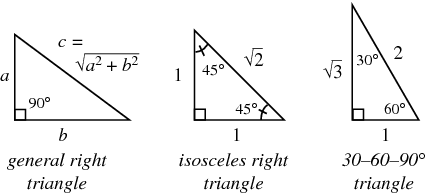



Right Triangle From Wolfram Mathworld




Special Right Triangles Video Lessons Examples And Solutions




30 60 90 Triangle Explanation Examples




30 60 90 Triangle Formulas Rules And Sides Science Trends



Solution I Dont Know How Do 30 60 90 Triangles That Already Have One Side And Also 45 45 90 Triangles And Sin 1 And All That Please Help
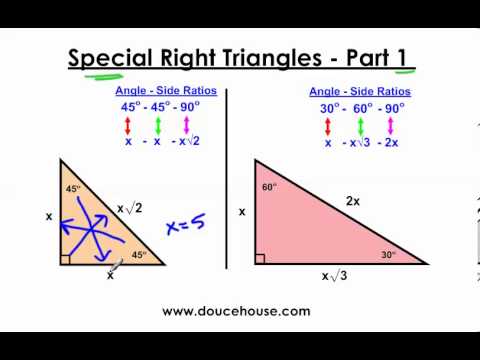



Special Right Triangles Part 1 45 45 90 And 30 60 90 Youtube




30 60 90 Triangle Formulas Rules And Sides Science Trends
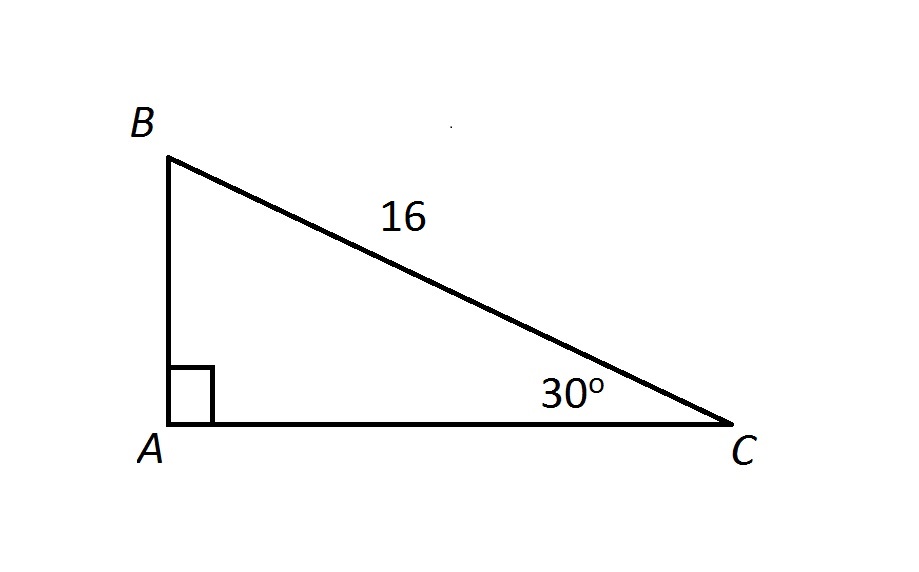



Special Triangles Hiset Math
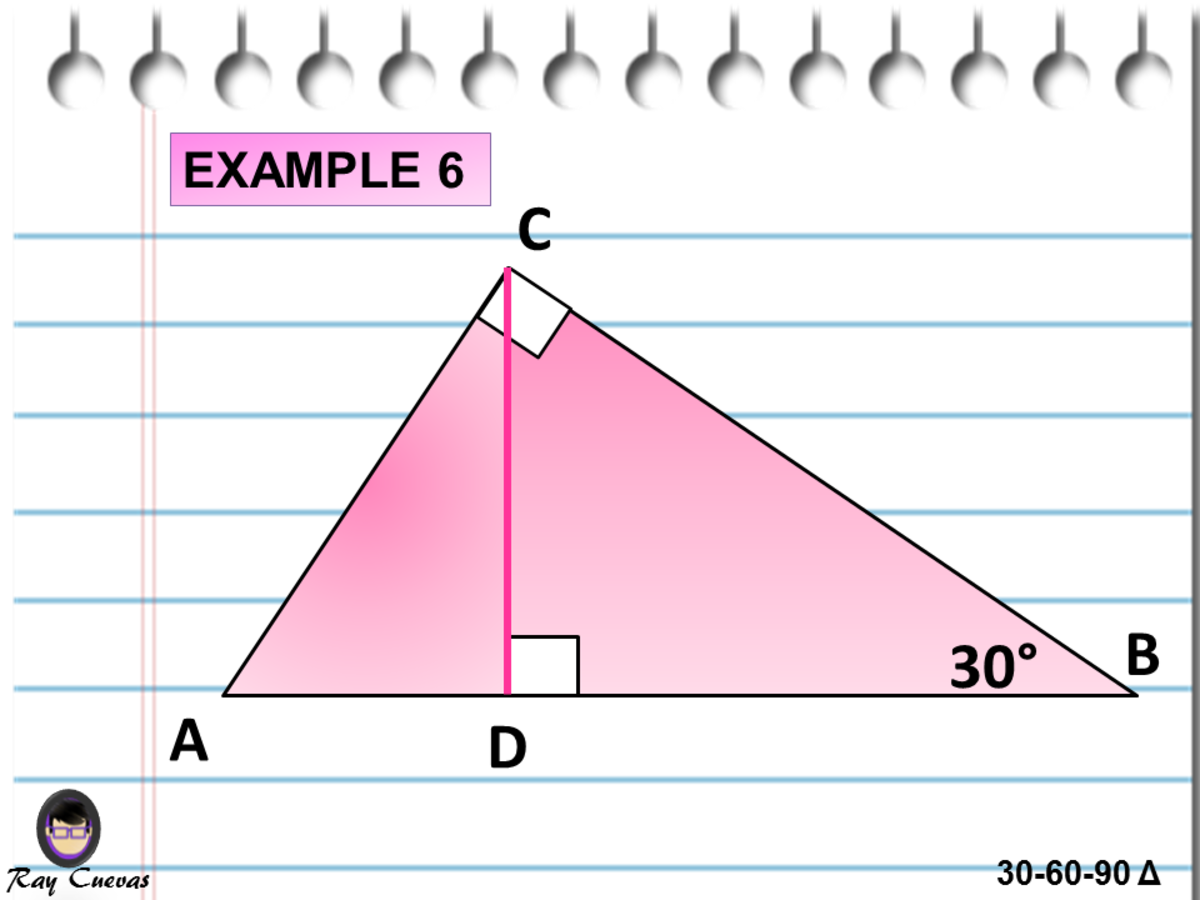



A Full Guide To The 30 60 90 Triangle With Formulas And Examples Owlcation




30 60 90 Triangle Definition Theorem Formula Examples




How To Work With 30 60 90 Degree Triangles Education Is Around
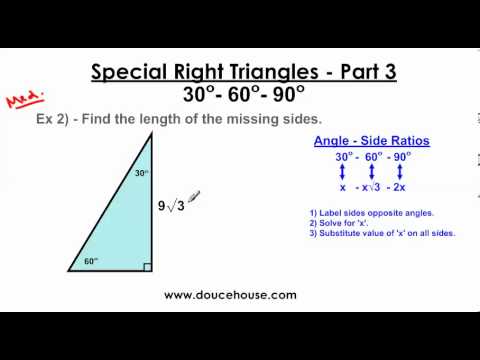



Special Right Triangles Part 3 30 60 90 Youtube




30 60 90 Triangle Theorem Properties Formula Video Lesson Transcript Study Com
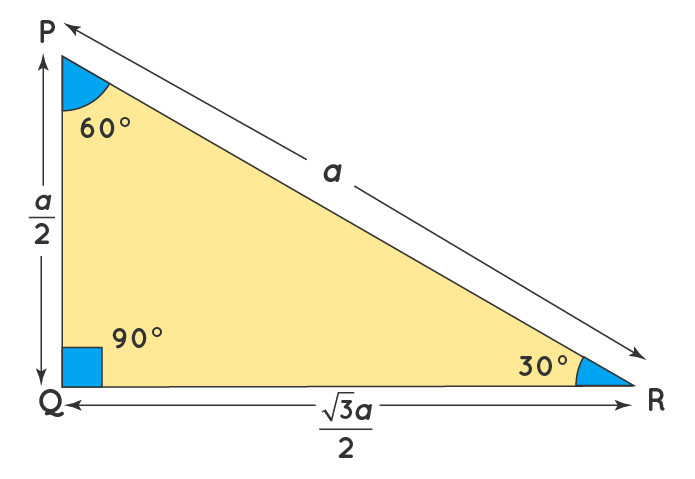



30 60 90 Triangle Definition Theorem Formula Examples
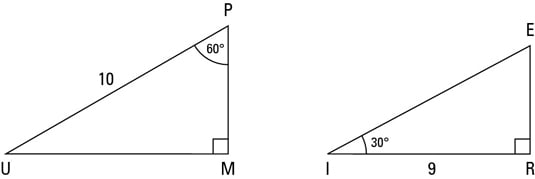



Identifying The 30 60 90 Degree Triangle Dummies




30 60 90 And 45 45 90 Triangles Youtube




30 60 90 Right Triangles Ck 12 Foundation




Relationships Of Sides In 30 60 90 Right Triangles Ck 12 Foundation




A Full Guide To The 30 60 90 Triangle With Formulas And Examples Owlcation




A Full Guide To The 30 60 90 Triangle With Formulas And Examples Owlcation




Instructions Use The Ratio Of A 30 60 90 Triangle To Solve For The Variables Leave Your Answers As Brainly Com




A Full Guide To The 30 60 90 Triangle With Formulas And Examples Owlcation
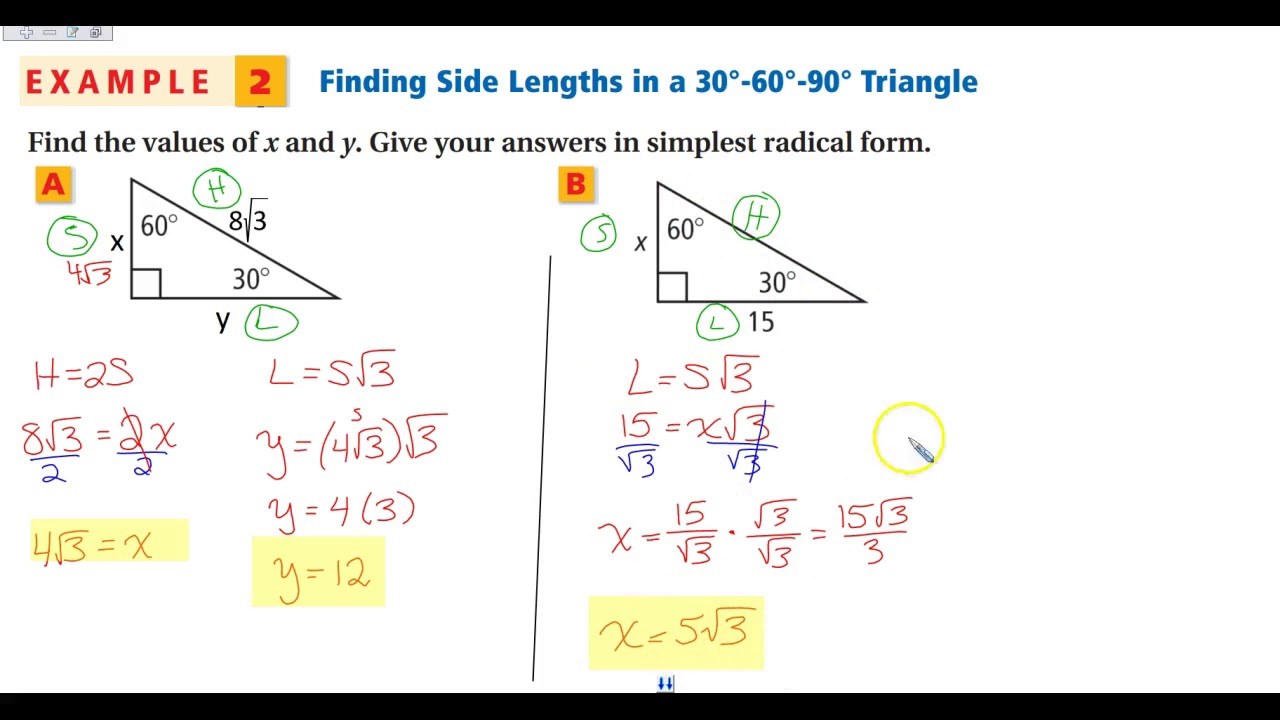



5 8b 30 60 90 Special Right Triangles Youtube




30 60 90 Triangles




The 30 60 90 Triangle Topics In Trigonometry




Act Set 6 Special Right Triangle Rules Flashcards Quizlet




The Complete Guide To The 30 60 90 Triangle



0 件のコメント:
コメントを投稿